|
the O. theorem
|
a group of works, 1999 - ongoing
|
|
|
|
|
background
|
the title of the group of works the O. theorem is inspired by ideas of the russian composer nikolai obukhov (1892–1954).
in 1982, after reading Gottfried Eberles essay "Absolute Harmonie" und "Ultrachromatik". Nikolaj Obuchov und Ivan Wyschnegradsky (published here), i started researching his work:
|
| around 1914, in the footsteps of scriabin, obukhov developed the idea of a “total harmony” (harmonie totale), wherein all twelve tones – in different octave ranges – are stacked into chord complexes. |
| the ban on the doubling of individual pitches led to the idea of a “vertical” twelve-tone music, remarkably different from the “horizontally” oriented one, in the legacy of schönberg. |
| obukhov himself did not implement this idea systematically in his œuvre. even his traité d’harmonie tonale, atonale et totale, published in 1947, contains only few examples of the “harmonie totale” in the corresponding section, as one harmonic method among many. |
| together with his "harmonie totale", obukhov developed a new notational system, which did away with the use of flats and sharps, and marked sharp notes by crosses inside the noteheads. |
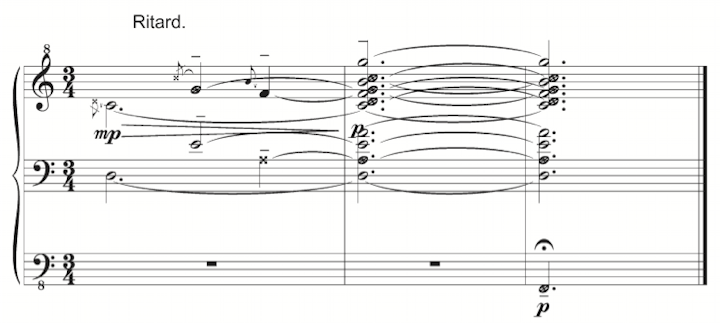 nikolai obukhov,
fragment du livre de vie (1914) | |
|
| sometimes obukhov also experimented with symmetrical interval constellations (the example constitutes, at the same time, a rare document of the unfolding of a symmetric chord as a “tone row”): |
 nikolai obukhov,
aimons-nous les uns les autres (1943) |
|
since 2015 i’ve been using the O. theorem as an umbrella term under which to subsume works (concepts, pieces & installations) from 1999 onwards, whos are connected by ideas in expandsion to obukhovs "harmonie totale". rather than the expressive and “mystical” connotations important for his œuvre, they focus on its conceptual and speculative potential:
a self-contained harmonic system in which every chord is an inversion of every other, and which can in principle also be translated to other divisions of the octave.
the possibilities range from clusters to centres of tonal gravity and abstract intervallic constructions. |
| because every pitch can only be used once, albeit in different octave positions, n^12 chords can be formed within n octaves (including possible transpositions). |
| this quickly reaches large dimensions, from 4096 possibilities within the range of two octaves up to 13,841,287,201 combinations in the range of seven octaves. |
apart from the 12-step equal temperament, the method can be extended, as a principle of a “permutative harmonics”, to arbitrary divisions of the tonal space, as long as two requirements are met:
1. a set (of tones) with a finite number of elements
2. the existence of a recursive principle, in analogy to octave identity (otherwise the set of pitches would automatically be equal to the chord). |
| filtering processes can also be used to carve out twelve-tone chords from (environmental) sounds: circle of fifths and clock of fifths, are works, that focus on the transfer of a music-theory space into a physical space and articulate this aspect of the O. theorem. |

